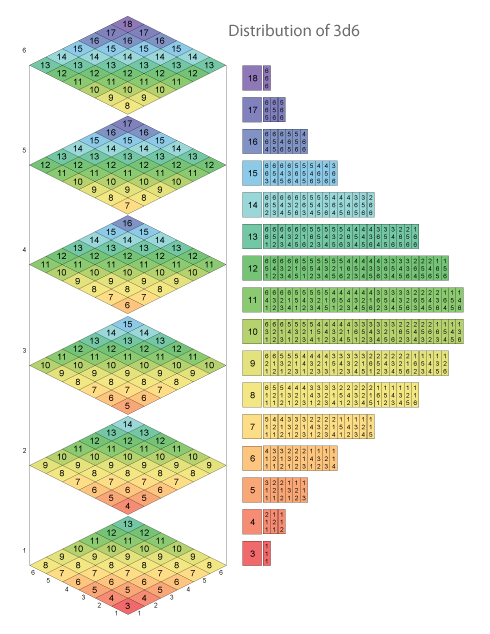
On the newly formed Praxis forum, user Keven Allen Jr asked:

I want a chart of every possible three die outcome of rolling 3d6 (order doesn’t matter)…. My mind doesn’t really love looking at numbers and percentages, but is very good at processing visual information….

As the notion of presenting probability information for role-playing games in a visual way dovetails with a possible future DivNull project, we dusted off the (rusty) graphic design tools and went to work (click for a larger version):

Direct download links:

• Large PNG (184 KB)
• Compressed SVG (11 KB)
• Adobe Illustrator source (407 KB)